Every country has a different currency rate and interest rate. When it comes to investment, the principle of interest rate parity helps you balance these two numbers. This is an in-depth examination of the interest rate parity formula and its significance.
You May like : Dow Theory Explained: What It Is and How It Works
Understanding the Dow Theory

An equation called interest rate parity (IRP) is used to control how interest rates and currency exchange are related to one another. Investors use it, and it is essential to the relationship between interest rates, foreign exchange rates, and spot exchange prices on the foreign currency markets.
The basic tenet of the IRP is that the difference in interest rates between two nations will match the difference between the forward and spot exchange rates. The hedged returns on your currency investments should remain constant despite changes in interest rates.
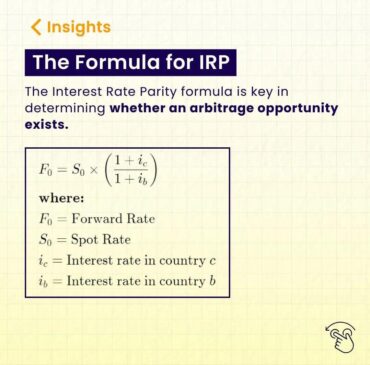
The forward exchange rate is determined by multiplying the spot currency exchange rate by the domestic interest rate and dividing the result by the interest rate in the foreign currency.
The concept of no-arbitrage is also based on interest rate parity. This is the buying and selling of a single asset in foreign exchange markets that allows a trader to profit from price differences. Stated otherwise, a foreign exchange trader cannot lock in a lower currency conversion rate from one nation while concurrently buying a higher interest rate currency from another.
Also Read : Investment Banking Trends to Watch in 2024
Interest Rate Parity (IRP) Excel Calculator
We will go through an example IRP inquiry below. Excel may be used to efficiently illustrate the computations necessary to determine the link between interest rates and the exchange rate of two nations.
Interpreting the Interest Rate Parity (IRP) Theory
The link between interest rates is seen in the diagram above. Assuming that currencies are in equilibrium, you can see how the interest rates and exchange rates should be held if you were to start at any corner. We can see what would happen if we were to exchange this money at the spot rate, invest at the foreign interest rate, and then exchange money back into the home currency, starting from the top left corner, if you were to borrow money at your home interest rate.
The amount you invested in the foreign interest rate, exchanged at the spot rate, and then exchanged at a later date should equal, in accordance with the IRP relationship, the amount you would have invested in the home currency interest rate for the same duration. According to the IRP theory, you shouldn’t be able to make money by just exchanging money if currencies are in equilibrium.
Why is Interest Rate Parity (IRP) Important?
The idea of interest rate parity is crucial. You may earn without taking any risks if the interest rate parity connection is broken. An arbitrage approach could be used in the event that IRP is not true. Let’s examine a situation where the forward and spot exchange rates are out of balance, for instance.
You may earn from arbitrage if the real forward exchange rate is greater than the IRP forward exchange rate. In order to accomplish this, you would borrow funds, lock in the forward contract, exchange them at the spot rate, and invest at the foreign interest rate. You would return the borrowed funds and swap the funds back into your native currency when the forward contract matured. You would have more than what you would have to repay if the forward price you locked in was higher than the IRP equilibrium forward price. All you have done with borrowed money is make essentially riskless money.

Complete Guide to GARP FRM' 25 Exam | Study Plan, Materials & Tips to Ace the Exam
Uncovered Interest Rate Parity vs Covered Interest Rate Parity
The interest rate parities of covered and uncovered bonds are extremely comparable. The distinction is that the state in which no-arbitrage is upheld without the need for a forward contract is referred to as the “uncovered IRP.” The projected exchange rate modifies in the uncovered IRP so that the IRP remains stable. The projected spot exchange rate calculation includes this idea.
When a forward contract is used and no-arbitrage is satisfied, the situation is referred to as covered interest rate parity. Because the forward exchange rate is keeping the currencies in balance, investors in the covered IRP would not care which interest rate to invest in—that of their own country or that of the foreign country. The determination of the forward exchange rate includes this idea.
What is the Interest Rate Parity (IRP) Equation?
The main distinction between the covered and uncovered IRP equations is that the forward exchange rate is used in place of the anticipated spot exchange rate. The formula for the uncovered interest rate parity is displayed as follows:
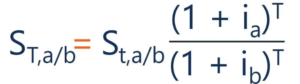
ST(a/b) = St(a/b) * (1+ ia) / (1 + ib)
The following is the equation for the covered interest rate parity:

Ft(a/b) = St(a/b) * (1+ ia)T / (1 + ib)T
Interest rate parity is also often shown in the form that isolates the interest rate of the home country:

For all forms of the equation:
- St(a/b) = The Spot Rate (In Currency A Per Currency B)
- ST(a/b) = Expected Spot Rate at time T (In Currency A Per Currency B)
- Ft(a/b) = The Forward Rate (In Currency A Per Currency B)
- ia = Interest Rate of Country A
- ib = Interest Rate of Country B
- T = Time to Expiration Date

